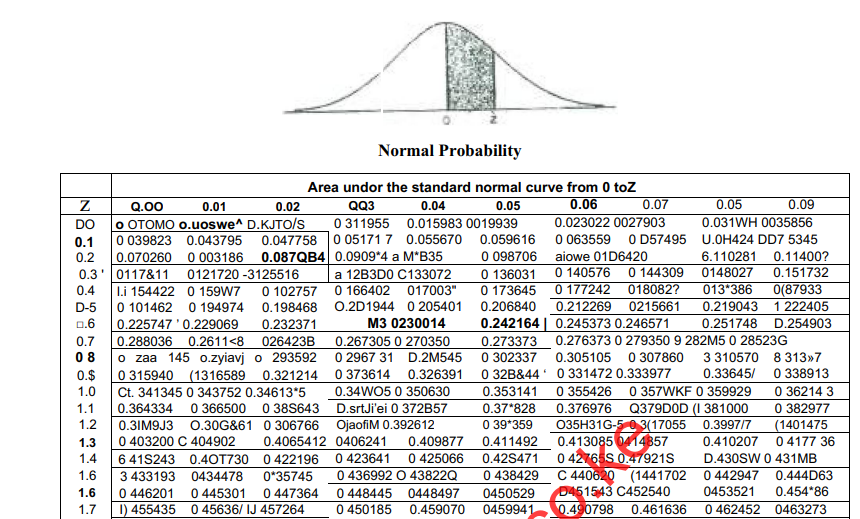
State one advantage and two disadvantages of presenting data in a distribution by grouping into classes, (3 marks)
table I shows the frequency distribution of the marks scored oul of 100 by 250 students of Octopus University during the second semester of 2010 academic year, Use it to answer the questions that follow.
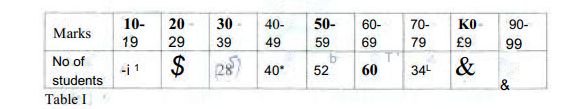
(i) Determine the marks which enclose the central 50% of the students. {4 marks)
Determine lhe proportion of students w ho would pass if the pass-mark is set at 45 marks. (4 marks)
(iii) The college requires the top 70% of the students to pass. Determine the pass-mark that should he set to achieve this. (3 marks)
(iv) A credit grade is awarded to marks in the range 55 to 75. Determine the number of students who would get a credit grade. (6 marks)
(a) Define the term time series as used in statistics. (2 marks)
(b) Using words and mathematical notations, explain the properties of the mean as applied in statistics. (8 marks)
(c) With the aid of a diagram in each case, illustrate the relative positions of the three measures of central tendency for each of the following types or distributions:
(i) symmetrical distribution;
(ii) negatively skewed distribution;
(ii) positively skewed distribution. (6 marks)
d Explain two limitations of the use of computers in statistical applicafmhs1 (4 marks)
(a) State four advantages and three disadvantages of the median as a statistical measure. (7 marks)
<b) Given a finite population of R40 sampling units, sclcci a random sample of size 20 using a systematic sampling technique Assume a random start at 647th unit
(c) The ittoitthly salaries of employees of a commercial bank with branches in Kenya and South Africa w ere analysed. The salaries of employees in Kenya were found to have a mean of Ksh 70.000 and a standard deviation of Ksh 12T
000. while the salaries of employees in South Africa were found to have a mean of 10,000 Rand and a standard deviation of 2.500 Rand
(i) Stale whether the measures used are appropriate for comparing the dispersion. If not then recommend the most suitable measure. Justify your answer (4 marks)
(ti) Identify the country which has a higher dispersion in income using the recommended measure in (i), (3 marks)
QI Differentiate between discrete data and con/muqw data giving two examples in each case (6 marks)
(b) Define the term critical path as used in project network analysis. (2 marks)
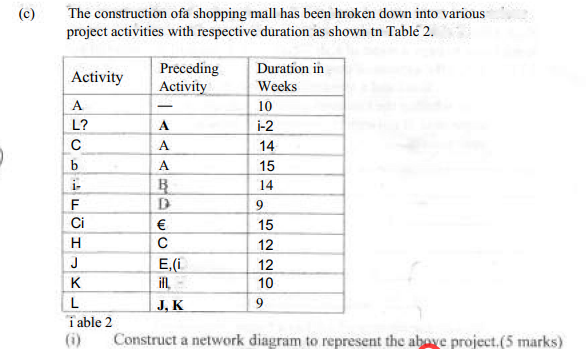
(ii) Determine the critical path of the network are the expected project duration. (4 marks)
(iii) Compute the total floats lor the non-critical activities. (3 marks)
6. (a) Distinguish between primary data and secondary data as used statistics. (4 marks)
(b) The Police Department collected data from a random sample of 10 PSV drivers on their driving experience in years and the corresponding number of traffic offences they committed in the year 2010. The data is as shown in

Determine the coefficient of determination between the number of traffic offences aid the length of experience of the PSV drivers.
(2 marks)
(iii) Draw conclusions from each of the measures computed in (i) and (ii) above, (6 marks)
(a) State three characteristics of the binomial probability distribution. (3 marks!
Define the term derivative of a function as used in statistics. (2 marks)
A private university analysed its past data and derived a profit function defined by the equation p< x) “ 21 x – 2v – 40. where x represents the enrolment of students in thousands and the constant 40 represents fixed costs.
Given that rhe profit and cost figures are in million Ksh, determine the following about the university;
(i) Break-even pomtfs) for student enrolment; (4 marks)
(ii) Enrolment of students that maximises profit and the corresponding profit. (3 marks)
(in) Suppose the university implements a new policy which is expected to reduce the fixed costs by 4 million Ksh. determine the new break-even point) for the student enrolment. (3 marks)
(c) Suite the application of each of the following tn financial mathematic!.;
(i) arithmetic progression;
(it) geometric progression. (2 marks)
(d) The Central) Bank issued bonds worth 10 million shillings redeemable after H years. Determine how much they should invest in sinking fund earning interest at a rate of 12% p.a. in order to he able to redeem the bond.