TUESDAY: 2 August 2022. Morning paper. Time Allowed: 3 hours.
Answer ALL questions. Marks allocated to each question are shown at the end of the question. Show ALL your workings. Do NOT write anything on this paper.
QUESTION ONE
1. In relation to statistics, distinguish between the following terms:
Primary data and secondary data. (2 marks)
Census inquiry and sample inquiry. (2 marks)
2. Highlight four properties of a good measure of central tendency. (4 marks)
3. The data below shows profits earned by XYZ company Ltd. in the years 2020 and 2021.
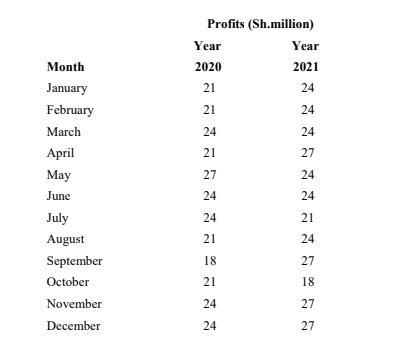
Required:
Create a table of cumulative totals and moving averages from the above data: (5 marks)
Construct a Z- chart to represent the above data using the table created in 3 (i) above. (5 marks)
Comment on the above graph with regard to profits earned by XYZ Company Ltd. (2 marks)
(Total: 20 marks)
QUESTION TWO
1. A small scale trader deposited Sh.2,000 in a bank account in the first month. The trader continued to increase the deposits by Sh.800 every other month until he deposited Sh.94,000 as the last amount.
Required:
The number of months until the last deposit was made. (3 marks)
The total amount in the bank account when the trader made the last deposit. (2 marks)
The amount deposited in the 30th month. (2 marks)
2. Mr. Kimani Memba, a salesman earns a basic salary and a commission on sales made.
In January 2022 he made sales amounting to Sh.200,000 and received total earnings of Sh.21,000.
In February 2022, he made sales worth Sh.300,000 and received total earnings of Sh.24,000.
Required:
Mr. Kimani’s basic salary and the rate of commission. (3 marks)
The total earnings Mr. Kimani would make on a monthly sales worth Sh.900,000. (1 mark)
The monthly sales made when his total earnings were Sh.36,000. (1 mark)
3. A manufacturer acquired a manufacturing machine at a cost of Sh.1,600,000. The estimated useful life of the machine is 5 years with a scrap value of Sh.400,000.
Required:
The annual rate of depreciation using the reducing balance method. (3 marks)
The difference between the net book value of the manufacturing machine after four years using reducing balance depreciation method and using the straight line depreciation method. (3 marks)
The value of the manufacturing machine after three years using reducing balance method. (2 marks)
(Total: 20 marks)
QUESTION THREE
1. James Oduor bought goods worth Sh.120,000. He projected to make a profit of 20% on the selling price.
Required:
The selling price of the goods. (4 marks)
2. Factorise the following expression:
12x2 – 13x + 1 (2 marks)
3. The total cost of 14 radios and 9 computers is Sh.458,000.
The total cost of 8 radios and 5 computers is Sh.256,000.
The items are later sold at a margin of 20% on each radio and 12% on each computer.
Required:
The cost of each radio and each computer using matrix algebra. (4 marks)
The selling price of each of the items in 3 (i) above. (2 marks)
4. Abex Limited invests in a particular project. The firm estimates that after x months of running, the cumulative profits in shillings (“000”) from the project is given by the function;
P = -x2 + 15x – 7
Where; x represents time in months.
The project can run for 20 months at the most.
Required:
The breakeven time in months for the project. (3 marks)
The quantity that maximises profits. (2 marks)
The total profits earned within the breakeven points. (3 marks)
(Total: 20 marks)
QUESTION FOUR
1. Explain the following terms as used in set theory:
Union of a set. (2 marks)
Intersection of a set. (2 marks)
Compliment of a set. (2 marks)
2. Distinguish between the following terms:
Additive rule and multiplicative rule as used in probability. (2 marks)
Permutations and combinations as used in counting techniques. (2 marks)
3. A recent survey was carried out on the types of the three latest fashionable hair styles most preferred by the ladies in Nairobi County namely; chemical use, braiding and hair cut. The survey that included 470 respondents revealed the following:
200 ladies preferred chemical use.
280 ladies preferred braiding.
190 ladies preferred hair cut.
90 ladies preferred chemical use and braiding.
70 ladies preferred chemical use and hair cut.
120 ladies preferred braiding and hair cut.
30 ladies preferred all the three hair styles.
Required:
Represent the above information in a Venn diagram. (4 marks)
The number of ladies who preferred none of the above hair styles. (4 marks)
The number of ladies who preferred only one type of hair style. (1 mark)
4. The number of ladies who preferred at least two types of the hair styles. (1 mark)
(Total: 20 marks)
QUESTION FIVE
1. Differentiate between “fixed base method” and “chain based method” as used in index numbers.(2 marks)
Explain four problems encountered in the construction of index numbers. (4 marks)
2. With reference to mean, mode and median, illustrate the following:
A positively skewed distribution. (2 marks)
A negatively skewed distribution. (2 marks)
3. 100 raffle tickets were sold at a certain entertainment venue in which 40 tickets were green in colour, 10 tickets were yellow and 50 tickets were blue.
Required:
Present the above information in a tree diagram. (4 marks)
The probability that the tickets chosen are of the same colour. (3 marks)
The probability that two tickets picked at random are yellow in colour. (3 marks)
(Total: 20 marks)
