
UNIVERSITY EXAMINATIONS: 2013/2014
ORDINARY EXAMINATION FOR THE BACHELOR OF SCIENCE
IN INFORMATION TECHNOLOGY
BIT 1206 DISCRETE MATHEMATICS
(DISTANCE LEARNING)
DATE: APRIL, 2014 TIME: 2 HOURS
INSTRUCTIONS: Answer Question ONE and any other TWO
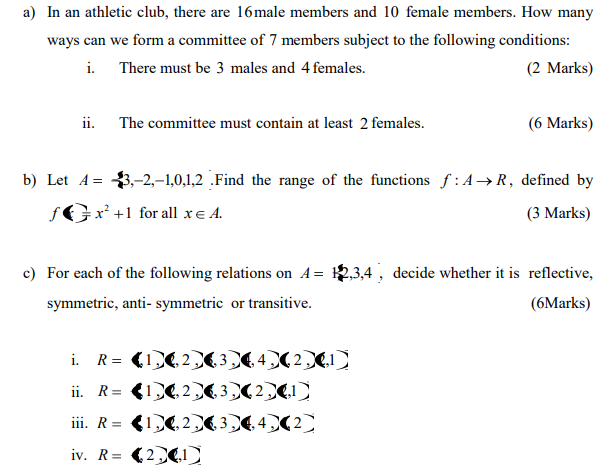
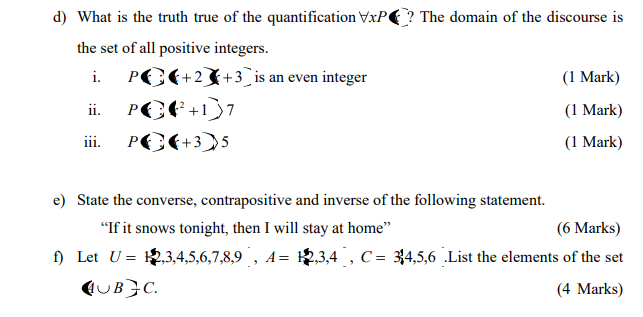
QUESTION ONE 🙁 30 MARKS)

QUESTION TWO :(20 MARKS)
a) Briefly describe a Hasse diagram. (3 Marks)
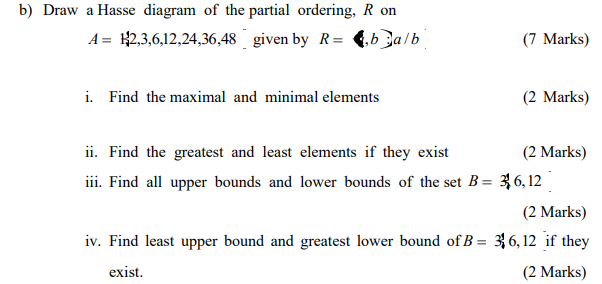
v. Is the poset a lattice? Give reason for your answer. (2 Marks)
QUESTION THREE: (20 MARKS)
a) Define the following terms
i. Literal (1 Mark)
ii. Minterm (1 Mark)
b) Prove the following Boolean law:
a a a
(3 Marks)
 s)
s)
QUESTION FOUR 🙁 20 MARKS)
a) Draw the graph,
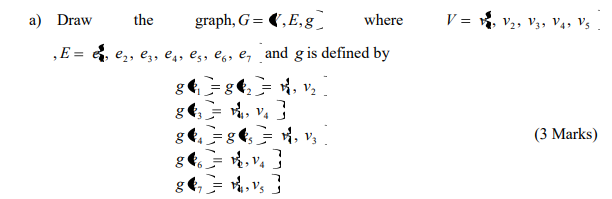
(3 Marks)
i. Find the degree of each vertex (5 Marks)
ii. Find all odd degree vertices (2 Marks)
iii. Find the adjacency matrix
AG
of the graph above (3 Marks)
iv. Find the incidence matrix
G
I
of the graph above (3 Marks)
v. Identify a pendant vertex of the graph above and give reason (2 Marks)
b) How many vertices are there in a graph with 15 edges if each vertex is of degree
3. (2 Marks)
QUESTION FIVE: (20 MARKS)
a) Find a SOP expansion of the following Boolean function using Boolean identities.

