
UNIVERSITY EXAMINATIONS: 2017/2018
EXAMINATION FOR THE CERTIFICATE IN BRIDGING MATHEMATICS
MAT 101: ALBEBRA & BUSINESS MATHEMATICS
DATE: DECEMBER, 2017 TIME: 1 ½ HOURS
INSTRUCTIONS: Answer Question ONE and any other TWO questions.
QUESTION ONE
a) The sums of the 6th and 17th terms of an Arithmetic Progression are 31 and 47 respectively.
Determine:
i) The first term, a (1 Marks)
ii) The common difference, d (3 Marks)
iii) The 40th term. (5 Marks)
iv) The sum to the 29th term. (5 Marks)
b) Solve for x
i) log1021 = log10 (3x-3) (4 Marks)
ii) log3
x+log3
3=log3
2 x+3 (4 Marks)

QUESTION TWO
a) Simplify the following expressions by rationalization

d) Using the quadratic formula, determine the roots to the equation:
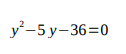
QUESTION THREE
a) By using factorization, determine the roots to the quadratic function:
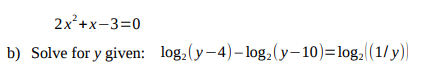
c) A father is four times as old as his son is. By 2037,the father will be 33 years older
than his son. Determine their respective years of birth. (4 Marks)
d) Solve the simultaneous equation
2 x+3 y−z=−5
x−4 y+2 z=21
5 x−2 y−3 z=−4 (8 Marks)
QUESTION FOUR
a) For the sequence -2, -3, 8, 11 …… , find:
i) The common difference, d (2 Marks)
ii) The fourth term. (2 Marks)
iii) The sum to the 80th term. (5 Marks)
e) Express in the form of a+b √ c (5 Marks)
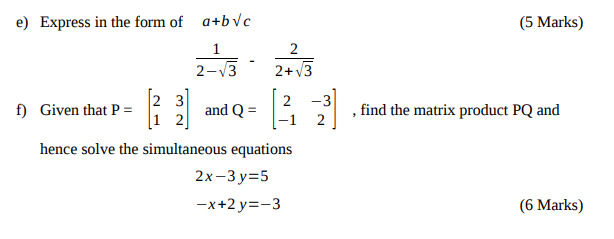
QUESTION FIVE
a) A credit union has issued a loan of Kshs. 600,800. Simple interest is charged
at the rate of 12% p.a. compute,
i) The interest accrued for the three year period (4 Marks)
ii) The total amount paid at the end of the 5 years period (5 Marks)
b) The value of machinery in a factory depreciates annually by 15%. If the machinery was valued at K£
57,500 after 3 years, calculate its original value (5 Marks)
c) Factorize the following functions and hence determine the values of the
variables x and y

