
2021 Mathematics Paper 1
Kenya Certificate of Secondary Education
Section I (50 marks)
Answer all the questions in this section.
1. An empty tank of capacity 18480 litres is to be filled with water using a cylindrical pipe of diameter 0.028 m. The rate of flow of water from the pipe is 2 m/s. Find the time in hours it would take to fill up the tank. (Take π = 22/7). (3 marks)
Vol. of water geting to the tank in 1sec
= 22/7 x 0.0142 x 2
= 0.001232 m3
Time needed to fill tank
= 18.48/0.001232
= 15000 sec
= 41/6 hours
2. The first term of a Geometric Progression (G.P) is 2. The common ratio of the G.P is also 2. The product of the last two terms of the G.P is 512. Determine the number of terms in the G.P. (3 marks)
nth term 2 x 2n-1
(n-1)th term = 2 x 2n-2
2 x 2n-1 x 2 x 2n-2=512
2n-1 = 9
2n-1 = 29
n = 5
3. The expression ax2 – 30x + 9 is a perfect square, where a is a constant. Find the value of a. (2 marks)
4 x a x 9 = (-30)²
a = 900/36
=25
4. Make x the subject of the formula y = bx OVER √cx2 – a5 (3 marks)
y² = b ²x ²/cx² – a
cx² – y² – ay² = b²x²
cx² – y²- b²x² = ay²
x² (cy² – b²) = ay²
x = ± √ ay ²/cy² – b²
5.The figure below shows a circle and a point outside the circle’.
Using a ruler and pair of compasses, construct a tangent to the circle from P. (4 marks)
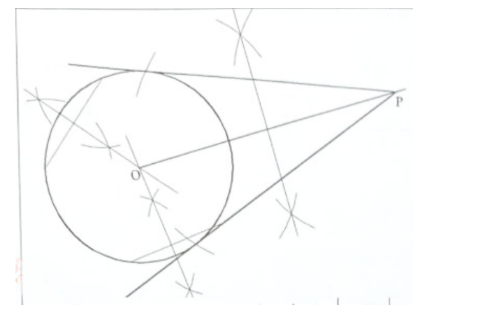
Locating centre O
⊥ bisector of OP
Arc showing the correct position of point of contact of circle and tangent
tangent drawn b utt
6. Four quantities P Q R and S are such that P varies directly as the square root of Q and inversely as the square of the difference of R and S. Quantity Q is increased by 44% while quantities R and S are each decreased by 10%.
Find the corresponding percentage change in P correct to 1 decimal place. (4 marks)
P = k √Q/(R – S)2
New value of Pafter changes in Q, R and S
= k √1.44Q
(0.9R – 0.9S)2
=1.481k √Q/(R – S)2
= (1.481 – 1)100
7. The figure below represents a prism ABCDEFGH of length 6 cm. The cross section BCFG of the prism is a trapezium in which GF = 11 cm, BC = 8 cm, BG = 5 cm and ∠GFC = ∠BCF = 90°
Calculate correct to 1 decimal place the angle between the line FA and the plane GFEH. (3 marks)
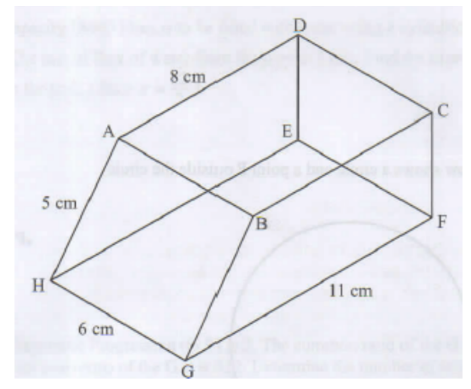
Let point A’ be the projection of point A on the plane GFEH AA’= √(5² – 3²) = 4 FA’ = √(6² + 8²) = 10 Tanθ = 4/10 = 0.4 θ =21.8°
8. The cash price of a gas cooker is Ksh 20 000. A customer bought the cooker on hire purchase terms by paying a deposit of Ksh 10 000 followed by 18 equal monthly instalments of Ksh 900 each, Annual interest, compounded quarterly, was charged on the balance for the period of 18 months. Determine, correct to 1 decimal place, the rate of interest per annum. (4 marks)
Balance upon paying deposit:
= 20000 – 10000
= 10000
Amount Repaid
= 900 x 18
= 16200
Let r =rate of interest per annum
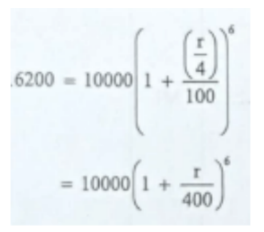
1 + r/400 =6√1.62 = 1.084
r = (1.084 – 1)x 400
= 33.6% or 33.5%
9. The table below shows the values oft and the corresponding values of h for a given relation
| t | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 |
|---|---|---|---|---|---|---|---|---|
| h | 8 | 4 | 2.7 | 2 | 1.6 | 1.3 | 1.1 | 1 |
a. On the grid provided, draw a graph to represent the information on the table given. (2
marks)
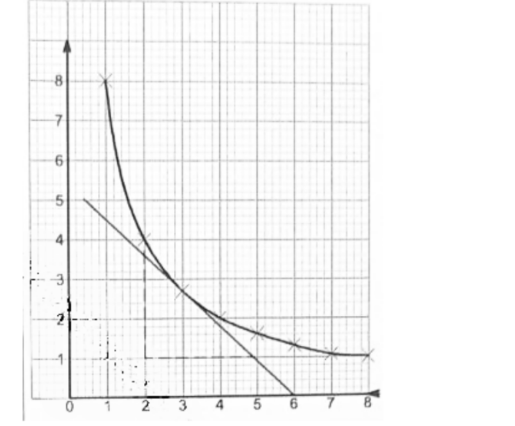
b. Use the graph to determine, correct to 1 decimal place, the rate of change of h at = 3. (2 marks)
Gradient = 0 – 27/6 – 3
= -0.9 ± 0.1
10. The equation of a trigonometric wave is y = 4 sin (ax – 70)o. The wave has a period of 180°.
a. Determine the value of a. (1 mark)
360/a – 180
a = 2
b. Deduce the phase angle of the wave. (1 mark)
Phase Angle = 70°
11. A point Q is 2000 nm to the West of a point P(40°N, 155°W). Find the longitude of Q to the nearest degree. (3 marks)
Let θ = longitude difference between P and Q
θ x 60 cos 40 = 2000
θ = 2000/60 cos 40
= 43.51°
= 155 + 43.51 = 198.51°
Longitude of Q
= 360° – 198.51°
161°E
180 – 18.51
= 161°E
12. A box contains 3 brown balls and 9 green balls. The balls are identical except for the colours. Two balls are picked at random without replacement.
a. Draw a tree diagram to show all the possible outcomes. (1 mark)
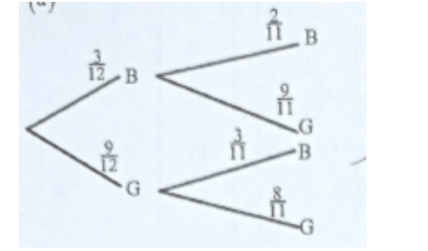
b. Determine the probability that the balls picked are of different colours. (2 marks)
P(Balls picked are of different colours)
=3/12 x 9/11 + 9/12 x 3/11
=27/132 + 27/132< p> =54/132
Accept 9/22
13. The figure below shows triangle XYZ.
Using a ruler and a pair of compasses locate point M is 2cm from line YX and is equidistant from lines YX and YZ. Measure length YM (3 marks)
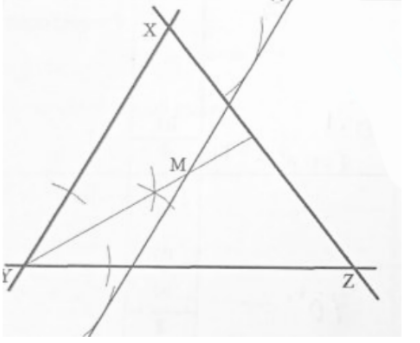
YM = (4 ± 0.1) cm
Angle bisector of ∠ XYZ
construction of a straight line 2 cm from and parallel to line XY
OW-1 if point M is not marked
14. The position vectors of points P, Q and Rare OP = 61-23 +3k, OQ = 121 – 5j + 6k and OR =8i-3j+4k. Show that P, Q and R are collinear points. (3 marks)

P is a common point
Points P, Q and R are collinear
15. In a transformation an object of area x cm2 is mapped on to an image whose area is 13x cm² Given that the matrix of the transformation is find the possible values of x. (3 marks)
3×2- 7(x – 1) – 13x/x = 13
3x² – 7x – 6 = 0
(3x + 2)(x – 3) = 0
x = -2/3 or x = 3
16. Find the area enclosed by the curve y=x2+2x the straight lines x = 1,x= 3 and the x-axis. (3 marks)
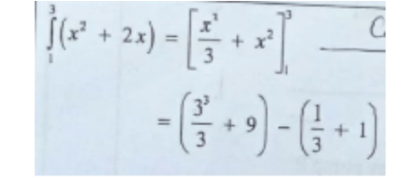
18 – 1⅓
= 16⅔ sq. units
SECTION II(50 mks)
Answer only five questions in this section in the spaces provided.
17. Pump P can fill an empty water tank in 7½ hours while pump Q can fill the same tank in 1¼ hours. On a certain day, when the tank was empty, both pumps were opened for 2½ hours.
a. Determine the fraction of the tank that was still empty at the end of the 2½ hours.(4 marks)
Fraction of tank filled by pumps P and Q in 1hr
= 1/7½ + 1/1¼ = 2 + 4
15 45
Fraction of tank filled by pumps P and Q in 2½ hrs
=2/9 x 5/2
=5/9
Fraction of tank still empty
= 1 – 5/9
=4/9
b. Pump P was later opened alone to completely fill the tank. Determine the time it took pump P to fill the remaining fraction of the tank.(2 marks)
Time taken by pump P alone to fill 4/9 of the tank
=4/9 ÷ 2/15
=4/9 x 15/2
= 3⅓hrs
c. The two pumps P and Q are operated by different proprietors. Water from the full tank was sold for Ksh 15 750.
The money was shared between the two proprietors in the ratio of the quantity of water supplied by each.
Determine the amount of money received by the proprietor of pump P. (4 marks)
Total time Pump P has pumped
= 2½+ 3⅓
= 5⅚ hrs
Fraction of tank delivered by pump P
=2/15 x 5⅚
=7/9
Amount received by propriator of Pump P
=7/9 x 15750
= Ksh 12 250
18. A rectangular plot measures 50 m by 24 m. A lawn, rectangular in shape, is situated inside the plot with a path surrounding it as shown in the figure below.
The width of the path in x m between the lengths of the lawn and those of the plot and 2x m between the widths of the lawn and those of the plot.
a. Form and simplify an expression in x for the area of the:
i. lawn; (2 marks)
Area of lawn = (50 – 4x)(24 – 2x)
= 1200 – 100x – 96x + 8x² = 1200 – 196x + 8x²
ii. path.(1 mark)
Area of path
= 50 x 24 – (1200 – 196x + 8×2)
= 1200 – 1200 + 1960 – 8×2
= 196x – 8×2
b. The area of the path is 1½ times the area of the lawn.
i. Form an equation in x and hence solve for X (4 marks)
196x – 8×2
=3/2(1200 – 196x + 8×2)
= 1800 – 294x + 12×2
= 20×2 – 490x + 180
= 2x² – 49x + 180 = 0
= (2x – 9)(x – 20) = 0
= x = 4.5 or x = 20
ii. Determine the perimeter of the lawn. (3 marks)
Length of lawn
= 50 – 4 x 4.5
= 32m
Width of lawn
= 24 – 2 x 4.5
= 15m
Perimeter of lawn
= 2(32 + 15)
= 94 m
19. In the figure below, points A, B, C, D and E lie on the circumference of a circle centre O. Line FAG is a tangent to the circle at A. Chord DE of the circle is produced to intersect with the tangent at F.
Angle FAE = 30°, ZEDC = 110° and 2 OCB = 55°.
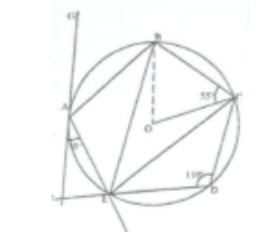
a. Determine the size of:
i. ∠ABC
Size of ∠AEC
∠ABE – 30°
(Angle in alternate segment)
∠CBE = 70°
(Opposite angle of a cyclic quadrilateral)
∠AEC – [180 – (30+70)] = 80°
(Opposite angle of a cyclic quadrilateral)
ii. ∠AEB. (3 marks)
∠BOC = 180-2×55 – 70°
∠BEC = 35°
Angle at the circumference is half angle at centre)
∠AEB – 80 – 35° = 45°
b. Given that AB-5 cm, ED -4.4 cm and FE = 2.5 cm. Calculate correct to 1 decimal place:
i. the radius of the circle. (2 marks)
Let radius of circle = R
2R = 5/sin 45°
R = 3.5 cm
ii. the length of line AF. (2 marks)
AF = √2.5 x (2.5 + 4.4)
AF = √17.25
= 4.2 cm
20. The table below shows income tax rates in a certain year.Monthly taxable income in Kenya shillings Tax rates
| Monthly taxable income in Kenya shillings | Tax rates |
|---|---|
| 0-12 298 | 10% |
| 12299-23 885 | 15% |
| 23 886-35 472 | 20% |
| 35 473 – 47 059 | 25% |
| 47 060 and above | 30% |
In the year, the monthly earnings of Kanini were as follows:
Basic salary : Ksh 64 500
House allowance : Ksh 12 000
Kanini contributes 7.5 % of her basic salary to a pension scheme. This contribution is exempted from taxation. She is entitled to a personal tax relief of Ksh 1 408 per month.
Calculate:
a. Kanini’s monthly taxable income.(2 marks)
Taxable income
= 64 500 + 12.000 – 7.5/100 x 64500
= Ksh 71662.50
b. the tax payable by Kanini that month. (6 marks)
Tax payable by Kanini
1st slab = 12798 x 10/100 = 1229.80
2nd slab = 11587 x 15/100 =1738.05
3rd slab = 11587 x 20/100 = 2317.4
4th slab = 11587 x 25/100 = 2896.75
5th slab = 24603 x 30/100 = 7381.05
Total tax = 15563.05
Tax less relief
= Ksh 15563.05 – 1408
= Ksh 14 155.05
c. Kanini’s net pay that month. (2 marks)
Total deductions
= 14155.05 + 75/100 x 64 500
= 18992.55
Net income =( 64500 + 12000) – 18 992.55
= 57 507.45
21. The vertices of the triangle shown on the grid are A’ (3,-3), B’ (1,-1) and C’ (3,-1).Triangle A’B’C’ is the image of triangle ABC under a transformation whose matrix is
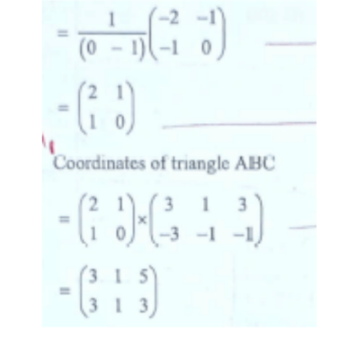
a. Find the coordinates of triangle A, B and C.(4 marks)
b. Triangle A”B”C” is the image of triangle A’B’C’ under a transformation matrix (2 marks)
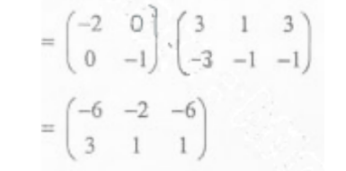
Coordinates of triangle ABC are A(3, 3), B(1,1) and C(5,3)
Determine the coordinates of A”, B” and C”.
c. On the same grid provided, draw triangles ABC and A”B”C”. (2 marks)
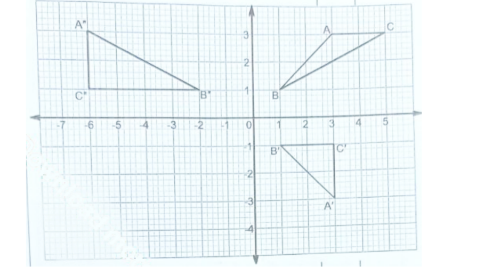
d. Determine a single matrix that maps ABC onto A”B”C”. (2 marks)

22. Workers in a factory commute from their homes to the factory. The table below shows the distances in kilometres, covered by the workers.
| Monthly taxable income in Kenya shillings | Tax rates |
|---|---|
| 0-12 298 | 10% |
| 12299-23 885 | 15% |
| 23 886-35 472 | 20% |
| 35 473 – 47 059 | 25% |
| 47 060 and above | 30% |
The mean distance covered was 14.5 km.
a. Determine the value of and hence the standard deviation of the distances correct to 2 decimal places. (6 marks)
| Midpoint x | f | xf | x2f |
|---|---|---|---|
| 3 | 3 | 9 | 27 |
| 8 | 6 | 48 | 384 |
| 13 | t | 13t | 1352 |
| 18 | 7 | 126 | 2268 |
| 23 | 4 | 92 | 2116 |
| 28 | 2 | 56 | 1568 |
| Σf = 22 + tΣxf = 331 + | 13tΣx | 2f = 7715 |
331 + 13t/22 + t = 14.5
Variance = √7715/30 – 14.5²
= 46.92
Standard deviation = √46.92
= 6.85
b. Calculate, correct to 2 decimal places, the interquartile range of the distances. (4 marks)
| UCB | 5.5 | 10.5 | 15.5 | 20.5 | 25.5 | 30.5 |
|---|---|---|---|---|---|---|
| C.F | 3 | 9 | 17 | 24 | 28 | 30 |
Q3 = 15.5 + 5.5/7 x 5
= 19.43
Q1 = 5.5 + 4.5/6 x 5
= 9.25
Interquartile range
Q3 – Q1 = 19.43 – 9.25
=10.18
23.a. Complete the table below giving the values correct to 1 decimal place.(2 marks)
| x | 30º | 90º | 150º | 210º | 300º | 330º |
|---|---|---|---|---|---|---|
|
2sin(¾x) – 2c
os2sin(¾x) |
1.1 | 2.6 | 2.6 | 1.1 | ||
| 1 + 2cos x | 2.7 | -0.7 | 2 |
Allow 7
B1 for any 5
b. On the grid provided and using the same axis, draw the graphs of y= 2sin(3⁄4x) -2cos(3⁄4x)and y=1+2cos x for 0o ≤ x ≤ 360°. (4 marks)

c. Using the graphs in part (b):
i. find the values of x for which sin(3⁄4x)= 1 + cos(3⁄4x) (2 marks)
When y=2
2sin(3⁄4x) – 2cos(3⁄4x)= 2 then
sin(3⁄4x) = 1 + sin(3⁄4x)
x = 120° and x = 240°
ii. determine the range of x for which 2sin(3⁄4x)-2cos(3⁄4x)>1 + 2 cos x (2 marks)
87° < x < 273
=± 2°
24. A particle was moving along a straight line. The acceleration of the particle after t seconds was given by (4t-13) ms-2. The initial velocity of the particle was 18 ms-1
a. Determine the value oft when the particle is momentarily at rest. (5 marks)
v = ∫(4t – 13)dt
= 2t2-13t+ c
when t = 0, v = 18
18 – 2 x 0 – 13 x 0 + c
c = 18
v = 2t2 – 13t + 18
When v-0
2t2 -13t + 18 = 0
= (2t – 9)(t – 2) = 0
t = 4.5 or t = 2
b. Find the distance covered by the particle between the time t = 1 second and t=3 seconds.(5 marks)
Distance covered by particle
Area above x axis
21∫(2t² – 13t + 18)dt
= [2/3t³ – 13/2t 2 + 18t]2 1
=[2 /3 x 2³-13/2 x 22 + 18 x 2] – [2/3 x 13 – 13/2 x 1² + 18 x 1]
=[16/3 – 26 + 36]-[2 /3 – 13/2 + 18]
= 151/3 – 121/6³ 1/6
Area below x axis
=[2/3 x 33 – 13/2 x 3² + 18 x 3] – 151 /3
=[18 – 117/2 + 54] – 151/3 = 15/6
Total area
= 31/6 + 15/6
= 5m
