
SECTION I (50 marks) Answer all the questions in this section in the spaces provided. 1. (a) Evaluate 540 396 — 726450/ 3 (l mark)
(b) Write the total value of the digit in the thousands place of the results obtained in (a) above. (1 mark)
2. Muya had a 62/3ha piece of land. He donated 7/8ha to a school and 11/2ha to a children’s home. The rest of the land was shared equally between his son and daughter. Find the size of land that each child got. (3 marks)
3. The volume of a cube is l728 cm3. Calculate, correct to 2 decimal places, the length of the diagonal of a face of the cube. (3 marks)
4. Use logarithms, correct to 4 significant figures, to evaluate

(4 marks)
5. A piece of Wire is bent into the shape of an isosceles triangle. The base angles are each 48° and the perpendicular height to the base is 6 cm. Calculate, correct to one decimal place, the length of the wire. (3 marks)
6. The density of a substance A is given as 13.6 g/cm’ and that of a substance B as 11.3 g/cm3. Determine, correct to one decimal place, the volume of B that would have the same mass as 50 cm3 of A. (3 marks)
7. Below is part of a sketch of a solid cuboid ABCDEFGH. Complete the sketch. (2 marks)
8. A salesman is paid a salary of Ksh 15 375 per month. He also gets a commission of 4½ on the amount of money he makes from his sales. In a certain month, he earned a total of Ksh 28 875. Calculate the value of his sales that month. (3 marks)
9. The sum of interior angles of a regular polygon is 24 times the size of the exterior angle.
(a) Find the number of sides of the polygon. (3 marks)
(b) Name the polygon. (1 mark)
10. The marks scored by a group of students in a test were recorded as shown in the table below.
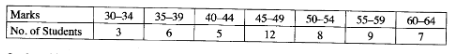
On the grid provided, and on the same axes, represent the above data using:
(a) a histogram; (3 marks)
(b) a frequency polygon. (1 mark)

ll. Given that P = 5a — 2b where a = and b = Find:
(a) column vector P; (2 marks)
(b) P’, the image of P under a translation vector (1 mark)
12. Given that a = 3, b = 5 and c =- ½ evaluate (3 marks)
13. The figure below represents the curve of an equation.

Use the mid-ordinate rule with 4 ordinates to estimate the area bounded by the curve, lines y=0,x= -3 and x=5. (3 marks)
14. The cost of 2 jackets and 3 shirts was Ksh 1 800. After the cost of a jacket and that of a shirt were increased by 20%, the cost of 6 jackets and 2 shirts was Ksh 4 800. Calculate the new cost of a jacket and that of a shin. (4 marks)
15. A tailor had a piece of cloth in the shape of a trapezium. The perpendicular distance between the two parallel edges was 30 cm. The lengths of the two parallel edges were 36 cm and 60 cm. The tailor cut off a semi circular piece of the cloth of radius 14cm from the 60 cm edge. Calculate the area of the remaining piece of cloth. (3 marks)
16. Musa cycled from his home to a school 6km away in 20 minutes. He stopped at the school for 5 minutes before taking a motorbike to a town 40km away. The motorbike travelled at 75 km/h. On the grid provided, draw a distance-time graph to represent Musa’s journey. (3 marks)
SECTION II(50 marks)
Answer any five questions in this section in the spaces provided.
17 Three partners Amina, Bosire and Karuri contributed a total of Ksh 4 800 000 in the ratio 4:5:7 to buy an 8 hectares piece of land. The partners set aside i of the land for social amenities and sub-divided the rest into 15 m by 25 m plots.
(a) Find:
(i) the amount of money contributed by Karuri; (2 marks)
(ii) the number of plots that were obtained. (3 marks)
(b) The puma: sokl the plots at Ksh 50000 each and spent 30% of the profit realised to pay for administrative costs. They shared the rest of the profit in the ratio of their contributions.
(i) Calculate the net profit realised. (3 marks)
(ii) Find the difference in the amount of the profit eamed by Amina and Bosire. (2 marks)
18. Two shopkeepers, Juma and Wanjiku bought some items from a wholesaler. Juma bought 18 loaves of bread, 40 packets of milk and 5 bars of soap while Wanjiku bought 15 loaves of bread, 30 packets of milk and 6 bars of soap. The prices of a loaf of bread, a packet of milk and a bar of soap were Ksh 45, Ksh 50 and Ksh 150 respectively.
(a) Represent:
(i) the number of items bought by Juma and Wanjiku using a 2 X 3 matrix. (1 mark)
(ii) the prices of the items bought using a 3 X 1 matrix. (1 mark)
(b)Use the matrices in (a) above to determine the total expenditure incurred by each person and hence the difference in their expenditure. (3 marks)
(c) Juma and Wanjiku also bought rice and sugar. Jurna bought 36 kg of rice and 23 kg of sugar and paid Ksh 8 160. Wanjiku bought 50 kg of rice and 32 kg of sugar and paid Ksh 11 340. Use the matrix method todetermine the price of one kilogram of rice and one kilogram of sugar. (5 marks)
19. Line AB drawn below is a side Of a triangle ABC.
![]()
(a) Using a pair of compasses and ruler only construct:
(i) triangle ABC in which BC = 10 cm and CAB = 90°; (2 marks)
(ii) a rhombus BCDE such that CBE = 120°; (2 marks)
(iii) a perpendicular from F, the point of intersection of the diagonals of the rhombus, to meet BE at G. Measure F G; (2 marks)
(iv) a circle to touch all the sides of the rhombus. (1 mark)
(b) Determine the area of the region in the rhombus that lies outside the circle. (3 marks)
20. In the figure below, AC = 12 cm, AD = 15 cm and B is point on AC. BAD = ADB = 30°.
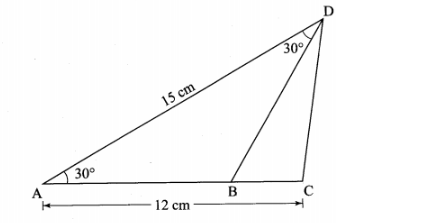
Calculate, correct to one decimal place:
(a) the length of CD; (3 marks)
(b) the length of AB; (3 marks)
(c) the area of triangle BCD; (2 marks)
(d) the size of £BDC. (2 marks) 21. (a) A straight line L1 whose equation is 3y -— 2x = -2 meets the x-axis at R. Determine the co-ordinates of R. (2 marks)
(b) A second line L2 is perpendicular to L] at R. Find the equation of L2 in the form y = mx + c, where m and c are constants. (3 marks)
(c) A third line L3 passes through (-4, 1) and is parallel to Ll. Find:
(i) the equation of L3 in the form y = mx + c, where m and c are constants. (2 marks)
(ii) the co-ordinates of point S, at which L3 intersects L2. (3 marks)
22. On the grid below, an object T and its image T’ are drawn.
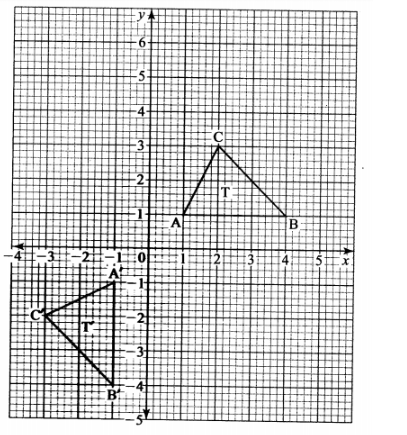
(a) Find the equation of the mirror line that maps T onto T’. (1 mark)
(i) T’ is mapped onto T” by positive quarter tum about (0, 0). Draw T”. (2 marks)
(ii) Describe a single transformation that maps T onto T”. (2 marks)
T” is mapped onto T”’ by an enlargement, centre (2, 0), scale factor -2. Draw T”’. (2 marks)
Given that the area of T’” is 12 cm’, calculate the area of T. (3 marks)
23. The figure below represents a conical flask. The flask consists of a cylindrical part and a frustum of a cone. The diameter of the base is 10 cm while that of the neck is 2 cm. The vertical height ofthe flask is 12 cm.
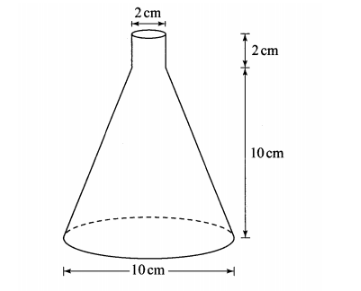
Calculate, correct to l decimal place:
(a) the slant height of the frustum part; (2 marks)
(b) the slant height of the smaller cone that was cut off to make the frustum part. (2 marks)
(c) the external surface area of the flask. (Take pie = 3.142) (6 marks)
24. The gradient of the curve
(a) Find:
(i) the value of p; (3 marks)
(ii) the equation of the tangent to the curve at x = 0.5.’ (4 marks)
(b) Find the co-ordinates of the turning points of the curve. (3 marks)
