
UNIVERSITY EXAMINATIONS 2017/2018
ORDINARY EXAMINATION FOR BACHELOR OF SCIENCE IN
INFORMATION TECHNOLOGY
BIT1110 MATHEMATICS FOR SCIENCE
BCU 102: FOUNDATIONS OF MATHEMATICS
BCT 1103: MATHEMATICS FOR ENGINEERS
BAC 1103: COMPUTING MATHEMATICS
(DAY/EVENING)
DATE: APRIL, 2018 TIME: 2 HOURS
INSTRUCTIONS: Answer question ONE and Any other TWO questions
QUESTION ONE: 30 MARKS (COMPULSORY)
a) Answer the following questions
i. State the remainder theorem

c) Four men and their wives sit on a bench. In how many ways can they be arranged if
i. There is no restriction 3 Marks
ii. Each man sits next to his wife 3 Marks
a) Find the third, tenth, twenty-first and nth terms of the A.P. with first term being 6 and
common difference 5. 4 Marks
d) Evaluate 2 sin 15o cos 15o 3 Marks
e) If you deposit KES 5000 into an account paying 6% annual interest compounded monthly,
how long until there is KES 8000 in the account? 4 Marks
f) Proof that 2 sin 0=1
2 Marks
QUESTION TWO: 20 MARKS
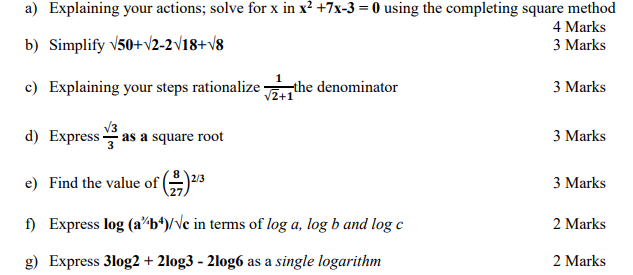
QUESTION THREE: 20 MARKS
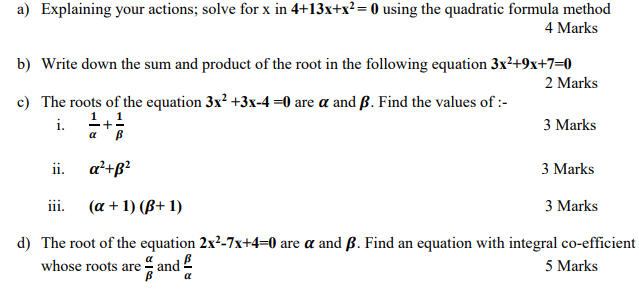
QUESTION FOUR: 20 MARKS
a) In an AP, the 13th term is 27, and the 10th term is three times the second term. Find the first
term, the common difference and the sum of the first ten terms 5 Marks
b) Find the third, tenth, twenty-first and nth terms of the G.P. which begins 3+6+…….
5 Marks
c) Triangle PQR, r = 5.75 and the sizes of angles P and Q are 42o
and 65o
respectively.
Calculate the length of PR 5Marks
d) In triangle PQR, QR = 3.5, RP = 4 and PQ = 5. Calculate the size of angle P and hence the
area of the triangle 5 Marks
QUESTION FIVE: 20 MARKS
a) On the graph paper provided plot the following graphs
![]()
a) Write 40 X 39 X 38 X 37 in factorial notation 3Marks
b) How many even numbers, greater than 2000, can be formed with the digits 1, 2, 4, 8, if each
digit may be used only once in each number? Explain your arguments. 4 Marks
c) Giving their forms, define the following terms 3 Marks
i. Irrational number
ii. Quadratic equation
iii. Polynomial expression
