
UNIVERSITY EXAMINATIONS 2017/2018
ORDINARY EXAMINATION FOR BSC IT, BSc. APPLIED COMPUTING, BBIT
BCT 1205/BAC 1202/BUSS 202/ BIT 1301: PROBABILITY AND STATISTICS
(DAY/EVENING/DISTANCE LEARNING)
DATE: AUGUST 2018 TIME: 2 HOURS
INSTRUCTIONS: Answer question ONE and Any other TWO questions
QUESTION ONE (30MARKS)
(a) These are Marks for 20 students in an assignment
84,17,38,45,47,53, 76,54,75,22,66,65,55,54,51,44,39,19,54,72
Draw a steam and leaf diagram to illustrate the data and determine the mode. (3Marks)
(b) The average height of 20 boys is 160cm, with a standard deviation of 4 cm. The average height of
30 girls is 155 cm, with a standard deviation of 3.5 cm. Find the standard deviation of the whole
group. (4 Marks)
(c) Find the median and the interquartile range of the following frequency distribution
X 5 6 7 8 9 10
F 6 11 15 18 6 5
(5Marks)
(d) Given that P(A)=
1
2
,P(A or B)=
3
5
.where A and B are events defined on a sample space S.
Determine P(B) given that (i) A and B are mutually exclusive
(ii) A and B are independent (6 Marks)
(e) In a beauty contest seven judges a warded two participants Jackie and Joan as follows

Calculate;
(i) The spearman’s rank correlation coefficient (4 Marks)
(ii) Comment on your results. (1 Mark)
(f) Digital limited is a manufacturing company that deals in the production of soft drink bottles.
During a quality assurance exercise, it was noted that of every production lot, 0.2 per cent of
soda bottles are found to be defective. The soda bottles are packed in crates each containing 25
bottles. A soft drinks manufacturer recently bought 1,500 crates of soda bottles from Digital
Limited. Using the Poisson distribution, determine the number of crates that will contain
(i) No defective soda bottle (3Marks)
(ii) At least two defective soda bottles (4Marks)
QUESTION TWO (20MARKS)
(a) Two identical baskets A and B contain white and red balls. Basket A contains 7 white balls and 3
red balls, while basket B contains 5 white balls and 5 red balls. A bag is chosen at random and 2
balls picked one after the other without replacement. Illustrate this information using a tree
diagram. (2Marks)
Find the probability that: –
(i) The two balls are of the same colour. (2Marks)
(ii) The two balls picked are of different colours. (2Marks)
(iii) Only one of the picked balls is red. (2Marks)
(iv) At least one white ball is picked. (2Marks)
(b) Let X be the number of shopping trips made during a given week by randomly selected family from
city. The following table lists the probability distribution.
X 0 1 2 3 4 5
P(X) 0.08 0.24 0.39 0.18 0.07 0.04
Calculate
(i) Does the distribution meet the two requirements for probability distribution of a
discrete random variable? Justify your answer. (3 Marks)
(ii) Mean of x (3 Marks)
(iii)Standard deviation of X (4 Marks)
QUESTION THREE (20MARKS
(a) The following data relates to annual profits in thousands of shillings made by an ICT firm
in different years
28 35 61 29 36 48 57 27
48 40 47 42 41 37 51 62
31 32 35 40 38 37 44 51
37 46 42 38 61 59 60 44
38 44 45 45 47 38 58 47
69 63 54 39 47 50 33 56
57 64
(i) Using a class interval of 10, construct a frequency distribution table for the data.
(4Marks)
(ii) Construct an Ogive and find the;
(a) Number of firms having profit between Ksh 37,000 and Ksh 58,000.
(b) Median profit (8 Marks)
(b)A recent article in the Business Daily listed the “Best Small IT Companies”. We are
interested in the current results of the companies ‘sales and earnings. A random sample of 8
companies was selected and the sales and earnings, in millions shillings are reported below.

Let sales be the independent variable and earnings the dependent variable.
Determine
(i) The regression equation (6Marks)
(ii) Estimate the earning for a small company with Sh.50 million in sales. (2Marks)
QUESTION FOUR (20 MARKS)
(a) A salesman makes a sale on the average to 40% 0f the customers he contacts. If four of
the customers are contacted today, what is the probability that he makes sales to exactly two?
(4Marks)
(b)A factory produces two types of electric lamps A and B, in an experiment relating to their
life, the following results were obtained.
Length of life( in
hours)
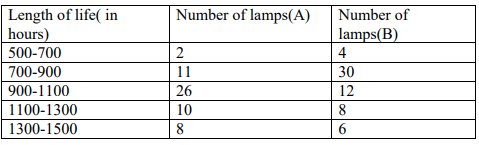
(i)Estimate the mean life for electric lamps A and B. (6Marks)
(ii) Estimate the standard deviation for electric lamps A and B. (6Marks)
(iii) Which electric lamp would you buy and why? (4Marks)
QUESTION FIVE (MARKS)
(a) Using illustrations explain four characteristics of the normal distribution. (8 Marks)
(b)The time taken by newspaper vendor to deliver the papers to an institute is normally
distributed with mean 12 minutes and standard deviation of 2 minutes. He delivers the papers
daily. Estimate the number of days during the year when he takes: –
(a) Longer than 17 minutes.
(b) Between 9 and 13 minutes.
(7Marks)
(c)The Marks of 500 IT students in an examination are normally distributed with a mean of 45
Marks and a standard deviation of 20 Marks. Given the pass mark is 40; estimate the number
of students who passed.
(5Marks)
