
MAASAI MARA UNIVERSITY
REGULAR UNIVERSITY EXAMINATIONS 2013/2014 ACADEMIC YEAR
THIRD YEAR FIRST SEMESTER
SCHOOL OF BUSINESS AND ECONOMICS
BACHELOR OF BUSINESS MANAGEMENT
COURSE CODE: BBM 350
COURSE TITLE: MANAGERIAL STATISTICS
DATE: 14TH APRIL, 2014 TIME: 2.00 – 5.00P.M.
INSTRUCTIONS TO CANDIDATES
Question ONE is compulsory
Answer any other THREE questions
This paper consists of 5 printed pages. Please turn over.
QUESTION 1: COMPULSORY, 30 MARKS,
a) Differentiate between the following statistical concepts
i. Descriptive statistics and inferential statistics (2 marks)
ii. Confidence level and significance level (2 marks)
iii. Point estimates and confidence interval (2 marks)
iv Continuous random variable and discrete random variable( 2 marks)
b) It is estimated that the old university bus breaks down 8 times in a week. Assuming that number of break downs follow a poisson distribution, find the probability that it breaks down ;
i. Exactly once per week (2 marks)
ii. At least thrice (3 marks)
c) The intelligence quotients (IQs) of 46 students from Loita showed a mean of 107 and a standard deviation of 10, while IQ of 30 students from Olkurto showed a mean of 112 and a standard deviation of 8. Is there a significant difference between IQs of the two groups at significance level of 0.05? ( 5 marks)
d) Three samples randomly selected from three independent populations that are normally distributed with equal variances produced the following data;

Test at 5% significance level whether the means of the three populations are equal ( 7 marks)
e) Prior to an advertising campaign, 35 per cent of a sample of 400 housewives used a certain product. After the campaign, 40 per cent of a second sample of 400 housewives used the same product. Did the campaign increase sales? ( 5 marks)
QUESTION 2
a) A child welfare officer asserts that the mean sleep of young babies is 14 hours a day. A random sample of 64 babies shows that the mean sleep was only 13 hours 30 minutes with standard deviation of 3 hours. At 5% level of significance, test the assertion that mean sleep of babies is less than 14 hours a day. ( 5 marks)
b) A traditional herb is claimed to be effective in curing colds. In an experiment on 328 people with colds, half of them were given the herb and half were given sugar pills. The patient’s reactions to the treatment are recorded in the following table;
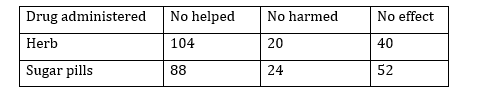
Test the hypothesis that the traditional herb is no longer better than sugar pills for curing Colds (10 marks)
c) sample of 100 motorcar tyres has a mean of 20,000 km and a standard deviation of 800 km. A second sample of 150 tyres has a mean life of 22,000 km and a standard deviation of 900 km. is it true to say that the two samples were drawn from the same population? ( 5 marks)
QUESTION 3
a) The breaking strengths of cables produced by manufacturer have a mean of 1800 Kg and a standard deviation of 100 Kg. by a new technique in the manufacturing process, it is claimed that breaking strength can be increased. To test this claim, a sample of 50 cables
is tested and it is found that the mean breaking strength is 1850Kg. can we support the claim at the 0.01 significance level? ( 5marks)
b) Two random samples were drawn from two normal populations and their values are;
A 66 67 75 76 82 84 88 90 92
B 64 66 74 78 82 85 87 92 93 95 97
Test whether the two populations have the same variance at 5 % level of significance
(10 marks)
c) A sales clerk in a departmental store claims that 60% of the shoppers entering the store leave without make a purchase. A random sample of 50 shoppers showed that 35 of them left without buying anything. Are the sample results consistent with the claim of the sales clerk?
Use a level of significance of 0.05 ( 5 marks)
QUESTION 4
a) Distinguish between type I and type II errors ( 6 marks)
b) Machine A and machine B produce identical components and it is required to test if the mean diameter of the components is the same. A random sample of 144 from machine A had a mean of 36.40mm and a standard deviation of 3.6mm, whilst a random sample of 225 from machine B had a mean of 36.90mm and a standard deviation of 2.9mm. Are the means significantly different at the 5% level? ( 6 marks)
c) The following set of data represents the age in years of newly married ladies in Ntulele; 15, 25, 17, 20, 22, 24, 19 and 16. This data was collected after the area chief made allegations that ladies in Ntulele get married at the age less than 18 years. Does this data provide enough evidence to support the claim at 5% level of significance? (8 marks)
QUESTION 5
a) A sample of 100 motor car tyres has a mean of 20,000 miles and a standard deviation of 800 miles. A second sample of 150 miles has a mean life of 22,000 miles and standard deviation of 900 miles. Is it true to say that the two samples were drawn from the same population? ( 5 marks)
b) A survey found that the average hotel room rate in Mombasa is KSh7, 500 and the average room rate in Nakuru is KSh6850. Assume that the data were obtained from two samples of 50 hotels each and that the standard deviations of the populations are KSh475 and KSh410 respectively. At α = 0.05, can it be concluded that there is a significant difference in the rates? ( 6 marks)
c) A random sample of 200 items from a production line selected for testing to estimate their average length of life. The sample mean was calculated to be 150 hours and the sample standard deviation found to be eight hours.
i. Calculate the 95% confidence interval for the population ( 4 marks)
ii. If it is required that estimation is carried out to within a maximum error of two percent, what minimum sample size should be selected at 95% confidence interval? ( 5 marks)
